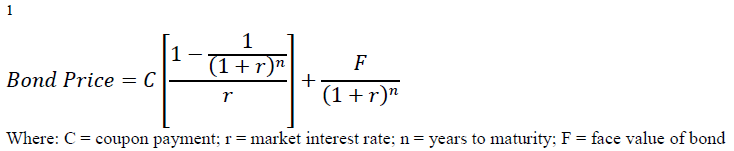In the previous two articles on Bonds, we talked about Investing in Bonds as an alternate investment instrument and the risks associated with investing in bonds. In this article, we will examine the relationship between bond prices and interest rates.

The bond market comprises a primary market and a secondary market. The primary market is where bonds are first issued by the borrowers (issuers) and purchased by (initial) investors. The secondary market is where investors sell their holdings of bonds and where subsequent buying and selling takes place with other investors. Investors who buy bonds do not have to hold them for the full term but may sell them in the secondary market through a stock broker on the stock exchange.

The value or price at which an investor can sell the bond in the secondary market will be determined by the current level of interest rates in the market compared to the coupon rate of the bond. The coupon rate is the fixed annual interest rate paid by the issuer to the bondholder for the entire term of the bond. Generally when interest rates across an economy fall, the price of bonds rises and vice versa. Thus, interest rates and bond prices move in opposite directions. The extent to which bond prices rise or fall depends on the magnitude of the interest rate movements. Bond price volatility will also vary because of its coupon and the term to maturity.

Let us look at an example of how interest rates affect bond prices. Suppose an investor buys $5,000 worth of Fiji Government bonds when it is first issued in the primary market by the Government. The bond has a fixed coupon rate of 10% and a life of 10 years i.e. it is a ten-year bond. Each year, for the next ten years, the investor will receive an annual interest payment of $500 (10% of $5,000). At the end of 10 years, Government (the issuer) will repay the investor the $5,000 it borrowed. The return or yield to maturity on the bond is 10%. The yield to maturity is the anticipated total return if the bond is held to the end of the term of its life.

To see how the market interest rates affect the price of bonds, let’s look at the example used above. The fixed coupon rate of 10% never changes even though the market interest rates may. Assume the investor wishes to sell the bond in the secondary market after four years, rather than holding it for the full term. We will look at two scenarios, one where interest rates have risen and one where interest rates have fallen.

Let’s say when the bond was first issued, similar bonds and other investments were also paying 10%. Therefore, the bond’s coupon rate is in line with the market and its price will be equal to face value. When the bond is selling at exactly its face value, the bond is said to be trading at par.

Rising Interest Rates

Assume that after four years, interest rates in the market have risen and similar 10-year bonds being issued at the time now pay a 12% coupon. The original bond is still paying 10% so it is now less attractive than other investments paying 12%. Investors will want to pay less for this bond if it is sold on the secondary market. This is why a bond’s price can be lower than its face or par value. In such instances, the bond is said to be trading at a discount.

Using a bond price formula1, the price of the bond will be calculated such that the annual interest payments of $500, plus the difference between what the new investor pays the bondholder and the face value of $5,000 to be received in 6 years, will result in a yield to maturity or return of 12% for the new investor over the remaining 6 years of the bond. The new investor will therefore buy the bond from the initial investor for $4,589 and receive a $500 coupon each year plus a principal repayment of $5,000 in the sixth year when the bond matures. The $500 per year plus the gain of $411 ($5,000 face value received less purchase price of $4589) will equate to a 12% per annum yield on the initial $4,589 invested by the new investor. The original bondholder, however, incurs a capital loss on his/her investment.

Falling Interest Rates

What happens if the market interest rate dropped to 8%? Now the bond is still paying a 10% coupon so it is more attractive than other investments paying only 8%. Investors will be willing to pay more for this bond. This is why a bond’s price can be higher than its face or par value, and in such instances the bond is said to be trading at a premium.

Because the bond pays an annual coupon of 10% which is higher than the current market rate of 8%, the price to be paid will be higher than the original price paid by the bondholder. The new investor would be required to pay $5,462 for the bond, which would yield him/her an annual return of 8%, equivalent to the current market rate. The original bondholder therefore makes a capital gain on his/her investment of $462.

In summary, a bond’s value (i.e. its price) and the interest rates in the market are inversely related.

So then the next question that arises would be what is the relevant interest rate for analysing a bond? Basically this should be the interest rate available on other investments that are similar to the bond (this is referred to as market yield). Why is this so? This is because if you don’t choose the bond, you can get this yield (interest rate) from other similar investments.

Therefore, the market yield is like the benchmark to measure a bond against. If the bond’s coupon rate isn’t as high as the market yield, investors will pay less than the face value. If the coupon rate is above the market yield, the bond is more attractive and investors will be prepared to pay more than the face value.

Finally, the prices of bonds with longer terms to maturity are likely to fluctuate more with rising interest rates than ones with shorter terms to maturity. The examples above illustrate that during the life of a bond, its capital value can change at any time in line with changes in the overall level of market interest rates. However, investors who hold the bonds to maturity are guaranteed that they will be repaid the face value of the bonds at maturity.